The concept of differential equations is closely related to differentiation. We can say that differential equation arrives from the field of differentiation. If you have a solid grasp of the field of differentiation and integration, differential equations won’t be very tough for you. An equation that consists of derivatives of the dependent variable with respect to the independent variables is called a differential equation. We can observe the presence of differential equations in a variety of fields which include physics, chemistry, economics, geology, anthropology, and so on. Thus, in-depth knowledge of this concept is of primal importance for the students. In this article, we will discuss the concept of differential equations with the help of examples. Before moving on to discuss differential equations, let us discuss in brief about differentiation.
What Do You Mean by the Term Differentiation?
We used the concept of differentiation when we need to measure the instantaneous rate of change in one of the functions based on its variables. In a layman’s language, the process that is used to obtain the derivative of any given function is known as differentiation. It is very important to study differentiation since it is a very broad sub-field of mathematics and has applications in almost all spheres of our lives. Before you set on learning about integration, learning and understanding the concepts of differentiation is a must.
Various Applications of Differentiation in Real-life
- Used in the field of business and finance to find out the break-out margin, profit and loss of a company, and so on.
- Widely used in the field of physics to find out various measurements.
- We use the concept of differentiation to measure temperature fluctuations.
What Do You Mean by the Term Differential Equation?
The best thing we can do to understand a concept is by checking out its examples first rather than the definitions. Let us take an example to understand the concept of differential equations clearly. Four equations are given below as follows:
- x3 – 73x + 52 = 0
- sin x + cos x + tan x + cosec x + cot x = 0
- x + y + 3 = 76
- x dy/dx + 36y = 0
Can you find an odd equation out of the four equations that have been given above? We can observe that the first three equations involve dependent variables and/or independent variables. However, the fourth equation i.e. x dy/dx + 36y = 0 consists of the variables and the derivative of the dependent variable with respect to the independent variable. The fourth equation given above is an example of a differential equation.
Various Types of Differential Equations
Generally, differential equations are classified into six types. Let us discuss all the types of differential equations briefly:
- Ordinary Differential Equation: Ordinary differential equation is an equation that has derivatives of the dependent variable w.r.t only one independent variable. Ordinary differential equations as the name suggests are one of the most common types of differential equations.
- Partial Differential Equation: Partial differential equation is an equation that has derivatives of the dependent variable w.r.t more than more independent variables.
- Homogeneous Differential Equation: Homogeneous differential equation is an equation that consists of equivalent degrees of the terms.
- Non-homogeneous Differential Equation: It is exactly the opposite of a homogeneous differential equation. A non-homogeneous differential equation is an equation that has different degrees of terms. A linear differential equation is one of the various types of non-homogeneous differential equations.
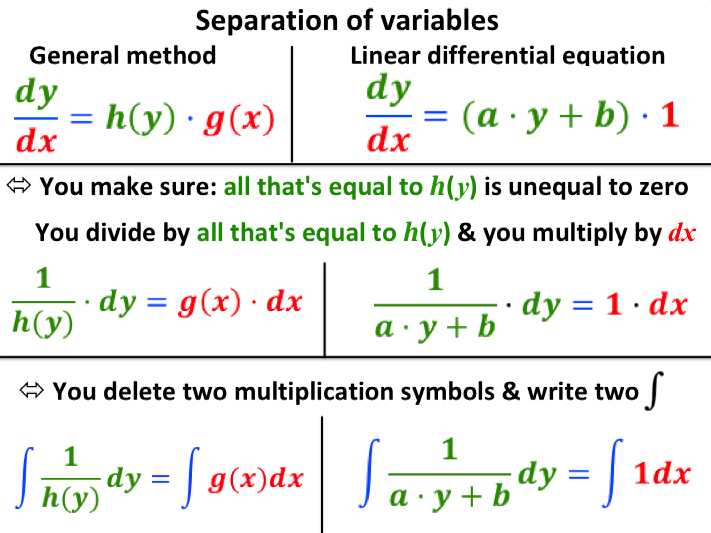
- Linear Differential Equation: A linear differential equation is an equation in which the different variables and their differentiation are only multiplied by constants.
- Non-linear Differential Equation: It is exactly the opposite of a linear differential equation. A non-linear differential equation is an equation that consists of non-linear terms.
If you want to learn more about these interesting concepts, visit Cuemath and introduce yourself to the whole new world of math where things are fun and interesting.




Be First to Comment